Do you want BuboFlash to help you learning these things? Or do you want to add or correct something? Click here to log in or create user.
Subject 4. Measures of Center Tendency
#has-images #quantitative-methods-basic-concepts #statistics
Measures of central tendency specify where data are centered. They attempt to use a typical value to represent all the observations in the data set.
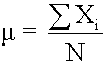
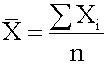
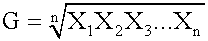
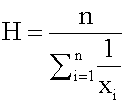
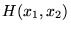
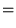
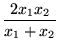
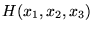
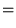
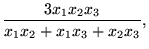
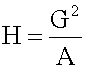
Population Mean
The population mean is the average for a finite population. It is unique; a given population has only one mean.
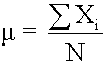
where:
- N = the number of observations in the entire population
- Xi = the ith observation
- ΣXi = add up Xi, where i is from 0 to N
Sample Mean
The sample mean is the average for a sample. It is a statistic and is used to estimate the population mean.
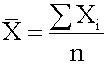
where n = the number of observations in the sample
Arithmetic Mean
The arithmetic mean is what is commonly called the average. The population mean and sample mean are both examples of the arithmetic mean.
- If the data set encompasses an entire population, the arithmetic mean is called a population mean.
- If the data set includes a sample of values taken from a population, the arithmetic mean is called a sample mean.
This is the most widely used measure of central tendency. When the word "mean" is used without a modifier, it can be assumed to refer to the arithmetic mean. The mean is the sum of all scores divided by the number of scores. It is used to measure the prospective (expected future) performance (return) of an investment over a number of periods.
- All interval and ratio data sets (e.g., incomes, ages, rates of return) have an arithmetic mean.
- All data values are considered and included in the arithmetic mean computation.
- A data set has only one arithmetic mean. This indicates that the mean is unique.
- The arithmetic mean is the only measure of central tendency where the sum of the deviations of each value from the mean is always zero. Deviation from the arithmetic mean is the distance between the mean and an observation in the data set.
The arithmetic mean has the following disadvantages:
- The mean can be affected by extremes, that is, unusually large or small values.
- The mean cannot be determined for an open-ended data set (i.e., n is unknown).
Geometric Mean
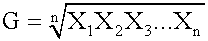
The geometric mean has three important properties:
- It exists only if all the observations are greater than or equal to zero. In other words, it cannot be determined if any value of the data set is zero or negative.
- If values in the data set are all equal, both the arithmetic and geometric means will be equal to that value.
- It is always less than the arithmetic mean if values in the data set are not equal.
It is typically used when calculating returns over multiple periods. It is a better measure of the compound growth rate of an investment. When returns are variable by period, the geometric mean will always be less than the arithmetic mean. The more dispersed the rates of returns, the greater the difference between the two. This measurement is not as highly influenced by extreme values as the arithmetic mean.
Weighted Mean
The weighted mean is computed by weighting each observed value according to its importance. In contrast, the arithmetic mean assigns equal weight to each value. Notice that the return of a portfolio is the weighted mean of the returns of individual assets in the portfolio. The assets are weighted on their market values relative to the market value of the portfolio. When we take a weighted average of forward-looking data, the weighted mean is calledexpected value.
Example
A year ago, a certain share had a price of $6. Six months ago, the same share had a price of $6.20. The share is now trading at $7.50. Because the most recent price is the most reliable, we decide to attach more relevance to this value. So, suppose we decide to "weight" the prices in the ratio 1:2:4, so that the current share price is twice as important as the price from six months ago, which in turn is twice as important as the price from last year.
The weighted mean would then be: (1 x 6 + 2 x 6.2 + 4 x 7.5) / (1 + 2 + 4) = $6.91. If we calculated the mean without weights, we'd get: (6 + 6.2 + 7.5) / 3 = $6.57. The fact that we've given more importance to the most recent (higher) share price inflates the weighted mean relative to the un-weighted mean.
Median
In English, the word "mediate" means to go between or to stand in the middle of two groups, in order to act as a referee, so to speak. The median does the same thing; it is the value that stands in the middle of the data set, and divides it into two equal halves, with an equal number of data values in each half.
To determine the median, arrange the data from highest to lowest (or lowest to highest) and find the middle observation. If there are an odd number of observations in the data set, the median is the middle observation (n + 1)/2 of the data set. If the number of observations is even, there is no single middle observation (there are two, actually). To find the median, take the arithmetic mean of the two middle observations.
The median is less sensitive to extreme scores than the mean. This makes it a better measure than the mean for highly skewed distributions. Looking at median income is usually more informative than looking at mean income, for example. The sum of the absolute deviations of each number from the median is lower than the sum of absolute deviations from any other number.
Note that whenever you calculate a median, it is imperative that you place the data in order first. It does not matter whether you order the data from smallest to largest or from largest to smallest, but it does matter that you order the data.
Mode
Mode means fashion. The mode is the "most fashionable" number in a data set; it is the most frequently occurring score in a distribution and is used as a measure of central tendency. A set of data can have more than one mode, or even no mode. When all values are different, the data set has no mode. When a distribution has one value that appears most frequently, it is said to be unimodal. A data set that has two modes is said to be bimodal.
The advantage of the mode as a measure of central tendency is that its meaning is obvious. Like the median, the mode is not affected by extreme values. Further, it is the only measure of central tendency that can be used with nominal data. The mode is greatly subject to sample fluctuations and, therefore, is not recommended for use as the only measure of central tendency. A further disadvantage of the mode is that many distributions have more than one mode. These distributions are called "multimodal."
Harmonic Mean
The harmonic mean of n numbers xi (where i = 1, 2, ..., n) is:
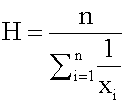
The special cases of n = 2 and n = 3 are given by:
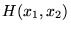
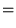
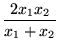
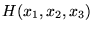
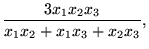
and so on.
For n = 2, the harmonic mean is related to arithmetic mean A and geometric mean G by:
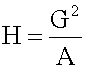
The mean, median, and mode are equal in symmetric distributions. The mean is higher than the median in positively skewed distributions and lower than the median in negatively skewed distributions. Extreme values affect the value of the mean, while the median is less affected by outliers. Mode helps to identify shape and skewness of distribution.
If you want to change selection, open original toplevel document below and click on "Move attachment"
Summary
| status | not read | reprioritisations | ||
|---|---|---|---|---|
| last reprioritisation on | suggested re-reading day | |||
| started reading on | finished reading on |
Details
Discussion
Do you want to join discussion? Click here to log in or create user.