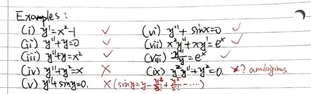Edited, memorised or added to reading queue
on 08-Nov-2023 (Wed)
Do you want BuboFlash to help you learning these things? Click here to log in or create user.
| status | not read | reprioritisations | ||
|---|---|---|---|---|
| last reprioritisation on | suggested re-reading day | |||
| started reading on | finished reading on |
| status | not read | reprioritisations | ||
|---|---|---|---|---|
| last reprioritisation on | suggested re-reading day | |||
| started reading on | finished reading on |
Flashcard 7596978867468
| status | not learned | measured difficulty | 37% [default] | last interval [days] | |||
|---|---|---|---|---|---|---|---|
| repetition number in this series | 0 | memorised on | scheduled repetition | ||||
| scheduled repetition interval | last repetition or drill |
The order of differential equation
The order of a differential equation is the highest derivative within the equation.
| status | not read | reprioritisations | ||
|---|---|---|---|---|
| last reprioritisation on | suggested re-reading day | |||
| started reading on | finished reading on |
| status | not read | reprioritisations | ||
|---|---|---|---|---|
| last reprioritisation on | suggested re-reading day | |||
| started reading on | finished reading on |
| status | not read | reprioritisations | ||
|---|---|---|---|---|
| last reprioritisation on | suggested re-reading day | |||
| started reading on | finished reading on |
Flashcard 7596995906828
| status | not learned | measured difficulty | 37% [default] | last interval [days] | |||
|---|---|---|---|---|---|---|---|
| repetition number in this series | 0 | memorised on | scheduled repetition | ||||
| scheduled repetition interval | last repetition or drill |
Classification of ODE by Linearity
Depending on whether the ODE has non-linear terms, the classification of the ODE are Linear ODE and Non-linear ODE.
Flashcard 7596997479692
| status | not learned | measured difficulty | 37% [default] | last interval [days] | |||
|---|---|---|---|---|---|---|---|
| repetition number in this series | 0 | memorised on | scheduled repetition | ||||
| scheduled repetition interval | last repetition or drill |
Classification of ODE by Linearity
Depending on whether the ODE has non-linear terms, the classification of the ODE are Linear ODE and Non-linear ODE.
Flashcard 7597005606156
| status | not learned | measured difficulty | 37% [default] | last interval [days] | |||
|---|---|---|---|---|---|---|---|
| repetition number in this series | 0 | memorised on | scheduled repetition | ||||
| scheduled repetition interval | last repetition or drill |
| status | not read | reprioritisations | ||
|---|---|---|---|---|
| last reprioritisation on | suggested re-reading day | |||
| started reading on | finished reading on |
Flashcard 7597018189068
| status | not learned | measured difficulty | 37% [default] | last interval [days] | |||
|---|---|---|---|---|---|---|---|
| repetition number in this series | 0 | memorised on | scheduled repetition | ||||
| scheduled repetition interval | last repetition or drill |
General Form for $2^{nd}$ Order Linear ODE
The general Form for \(2^{nd}\) Order Linear ODE is \(a(x)\frac{d^{2}y}{dx^{2}}+b(x)\frac{dy}{dx}+c(x)y=d(x)\) where \(a(x),b(x),c(x),d(x)\) are different function of \(x\).
| status | not read | reprioritisations | ||
|---|---|---|---|---|
| last reprioritisation on | suggested re-reading day | |||
| started reading on | finished reading on |
Flashcard 7597021596940
| status | not learned | measured difficulty | 37% [default] | last interval [days] | |||
|---|---|---|---|---|---|---|---|
| repetition number in this series | 0 | memorised on | scheduled repetition | ||||
| scheduled repetition interval | last repetition or drill |
Definition of the homogeneous ODE
The homogeneous ODE is the ODE which has no terms with a pure function of \(x\).
| status | not read | reprioritisations | ||
|---|---|---|---|---|
| last reprioritisation on | suggested re-reading day | |||
| started reading on | finished reading on |
Flashcard 7597025529100
| status | not learned | measured difficulty | 37% [default] | last interval [days] | |||
|---|---|---|---|---|---|---|---|
| repetition number in this series | 0 | memorised on | scheduled repetition | ||||
| scheduled repetition interval | last repetition or drill |
Definition of the inhomogeneous ODE
The inhomogeneous ODE is the ODE which has at least one term with a pure function of \(x\).
| status | not read | reprioritisations | ||
|---|---|---|---|---|
| last reprioritisation on | suggested re-reading day | |||
| started reading on | finished reading on |
Flashcard 7597028936972
| status | not learned | measured difficulty | 37% [default] | last interval [days] | |||
|---|---|---|---|---|---|---|---|
| repetition number in this series | 0 | memorised on | scheduled repetition | ||||
| scheduled repetition interval | last repetition or drill |
Classification of ODE by Homogeneity
Depending on whether it has a pure function of non-variable function \(x\), the classification of the ODE is the homogeneous ODE(Having no non-variable function) and inhomogeneous ODE(Having at least one non-variable function).
| status | not read | reprioritisations | ||
|---|---|---|---|---|
| last reprioritisation on | suggested re-reading day | |||
| started reading on | finished reading on |
Flashcard 7597038374156
| status | not learned | measured difficulty | 37% [default] | last interval [days] | |||
|---|---|---|---|---|---|---|---|
| repetition number in this series | 0 | memorised on | scheduled repetition | ||||
| scheduled repetition interval | last repetition or drill |
Principle of Superposition for ODE's solutions
If an ODE is linear and homogeneous, then the "Principle of Superposition" appears: If \(y_{1}\) and \(y_{2}\) both solve an ODE, then, \(y_{3}=\alpha y_{1}+\beta y_{2}\) is also a solution.
Flashcard 7597039947020
| status | not learned | measured difficulty | 37% [default] | last interval [days] | |||
|---|---|---|---|---|---|---|---|
| repetition number in this series | 0 | memorised on | scheduled repetition | ||||
| scheduled repetition interval | last repetition or drill |
Principle of Superposition for ODE's solutions
If an ODE is linear and homogeneous, then the "Principle of Superposition" appears: If \(y_{1}\) and \(y_{2}\) both solve an ODE, then, \(y_{3}=\alpha y_{1}+\beta y_{2}\) is also a solution.
| status | not read | reprioritisations | ||
|---|---|---|---|---|
| last reprioritisation on | suggested re-reading day | |||
| started reading on | finished reading on |
Flashcard 7597043354892
| status | not learned | measured difficulty | 37% [default] | last interval [days] | |||
|---|---|---|---|---|---|---|---|
| repetition number in this series | 0 | memorised on | scheduled repetition | ||||
| scheduled repetition interval | last repetition or drill |
General Form for 2nd Oder Linear Homogeneous ODE
The general form of \(2^{nd}\) order linear homogeneous ODE is \(a_{2}(x)\frac{d^{2}y}{dx^{2}}+a_{1}(x)\frac{dy}{dx}+a_{0}(x)y(x)=0\) where \(a_{n}(x),\ n=0,1,2\) are the function of \(x\).
| status | not read | reprioritisations | ||
|---|---|---|---|---|
| last reprioritisation on | suggested re-reading day | |||
| started reading on | finished reading on |
Flashcard 7597047549196
| status | not learned | measured difficulty | 37% [default] | last interval [days] | |||
|---|---|---|---|---|---|---|---|
| repetition number in this series | 0 | memorised on | scheduled repetition | ||||
| scheduled repetition interval | last repetition or drill |
Flashcard 7597049122060
| status | not learned | measured difficulty | 37% [default] | last interval [days] | |||
|---|---|---|---|---|---|---|---|
| repetition number in this series | 0 | memorised on | scheduled repetition | ||||
| scheduled repetition interval | last repetition or drill |
| status | not read | reprioritisations | ||
|---|---|---|---|---|
| last reprioritisation on | suggested re-reading day | |||
| started reading on | finished reading on |
Flashcard 7597053578508
| status | not learned | measured difficulty | 37% [default] | last interval [days] | |||
|---|---|---|---|---|---|---|---|
| repetition number in this series | 0 | memorised on | scheduled repetition | ||||
| scheduled repetition interval | last repetition or drill |
Mathematical Definition of Euler's Equation
欧拉公式提出,对任意实数\(x\),都存在\(e^{ix} = \cos x + i\sin x\),其中\(e\)是自然对数的底数,\(i\)是虚数单位,而\(\cos\)和\(\sin\)则是余弦、正弦对应的三角函数,参数\(x\)则以弧度为单位
| status | not read | reprioritisations | ||
|---|---|---|---|---|
| last reprioritisation on | suggested re-reading day | |||
| started reading on | finished reading on |
Flashcard 7597061180684
| status | not learned | measured difficulty | 37% [default] | last interval [days] | |||
|---|---|---|---|---|---|---|---|
| repetition number in this series | 0 | memorised on | scheduled repetition | ||||
| scheduled repetition interval | last repetition or drill |
Euler's Equation in Complex number set
由于该公式在\(x\)为复数时仍然成立,所以也有人将这一更通用的版本称为欧拉公式:\(e^{iθ} = \cosθ + i\sin θ \iff e^θ = \cosh θ + \sinh θ\)
| status | not read | reprioritisations | ||
|---|---|---|---|---|
| last reprioritisation on | suggested re-reading day | |||
| started reading on | finished reading on |
Flashcard 7597065637132
| status | not learned | measured difficulty | 37% [default] | last interval [days] | |||
|---|---|---|---|---|---|---|---|
| repetition number in this series | 0 | memorised on | scheduled repetition | ||||
| scheduled repetition interval | last repetition or drill |
The solution method for 2nd order linear homogeneous ODE
> The general form of 2nd-order linear homogeneous ODE is \(a_{2}(x)\frac{d^{2}y}{dx^{2}}+a_{1}(x)\frac{dy}{dx}+a_{0}(x)y(x)=0\) If \(a_{2}(x),a_{1}(x),a_{0}(x)\) are constant functions: 1. Try ansatz: \(y=e^{mx}\). 2. To find \(m\), plug \(y = e^{mx}\) into ODE. Rearrange the equation and get the auxiliary equation: \(\underbrace{(am^{2}+bm+c)}_{\text{Auxiliary Equation}}e^{mx}=0\) 3. Using the solution of the auxiliary equation to solve the ODE. <span>
| status | not read | reprioritisations | ||
|---|---|---|---|---|
| last reprioritisation on | suggested re-reading day | |||
| started reading on | finished reading on |
Flashcard 7597070617868
| status | not learned | measured difficulty | 37% [default] | last interval [days] | |||
|---|---|---|---|---|---|---|---|
| repetition number in this series | 0 | memorised on | scheduled repetition | ||||
| scheduled repetition interval | last repetition or drill |
The relations between the solution of an ODE and the solution of the ODE's auxiliary equation
Depending on the solution of the auxiliary equation, the solution of the ODE is different: \(m=\frac{-b\pm\Delta}{2a}, \Delta\equiv b^{2}-4ac\) 1. \(\Delta>0\Rightarrow\) 2 real roots: \(m_{1},m_{2}\), both \(y_{1}=e^{m_{1}x},y_{2}=e^{m_{2}x}\) works. So the general solution is \(y_{general}=Ae^{m_{1}x}+Be^{m_{2}x}\) - Alternative form: Because of the Euler's Equation in \(\mathbb{C}\), \(y(x)=e^{px}[\tilde{A}\cosh(qx)+\tilde{B}\sinh(qx)]\), where \(p,q\) are \(\displaystyle m=\underbrace{- \frac{b}{2a}}_p\pm \underbrace{\frac{\sqrt{\Delta}}{2a}}_{q}\). - Why we need this form? When the initial condition includes \(y(0)\), the alt form would be much easier 2. \(\Delta<0\Rightarrow\) 2 complex roots: \(m_{1},m_{2}\in\mathbb{C}\), so the general solution is \(y(x)=Ce^{m_{1}x}+De^{m_{2}x}\) - Alternative form: Because of the Euler's Equation, \(y(x)=e^{m_{r}x}[\tilde{C}\cosh(m_{i}x)+\tilde{D}\sinh(m_{i}x)]\), where \(m_{1,2}=m_{r}\pm im_{i}\). 3. \(\Delta=0\Rightarrow\) one \(m\) only! so the general solution is \(y(x)=Ae^{mx}+Bxe^{mx}=(A+Bx)e^{mx}\)